Geology 319 Structural Geology: The Architecture of Earth’s Continental Crust

Study Guide :: Unit 5

Folds: Waves of Solid Rock

“It is almost impossible to view waves of solid rock without wondering how materials we regard as strong can be folded in a manner that makes them seem so weak.” (Davis, Reynolds, & Kluth, p. 344)

Overview

Folds are very common structures in orogens, but may not be obvious on a scenic drive along a road through a mountain range. You might notice that beds dip to one direction or another in a particular mountain, but because of erosion, you may not see the closure, or hinge, of the fold. Nonetheless, dipping beds are the norm in orogens, and folding is the main cause. Folds occur over a wide range of sizes, from microscopic in some metamorphic rocks, to tens of kilometres across, such that an entire mountain range may be part of a single fold.

Understanding folds is important for interpreting the history of orogens, but folds have economic importance as well. Folds can control the location of petroleum deposits, and some mineral deposits form in fold cores as a result of hydrothermal (hot groundwater) precipitation. During folding, the layers of rock being folded can become separated in the hinge area, leaving open spaces in which mineral deposits can form.

In this unit on folds, Lesson 1 presents a mechanical perspective on what happens to rocks when they fold. Lesson 2 covers terminology related to folds. Lesson 3 explains how to describe the orientation of folds, and outlines a naming system for folds based on their orientations. Lesson 4 illustrates how to identify folds in outcrops and on maps, and Lesson 5 covers the ways in which folds can affect topography.

Objectives

After studying this unit, you should be able to

1. explain the different conditions under which folds form, and describe the mechanisms responsible.
2. explain when you would use the terms anticline, syncline, antiform, and synform.
3. identify the main parts of a folded surface.
4. use the system of fold classification to describe the orientation of a fold.
5. identify a fold in map view, and use information such as the relative age of strata and stratigraphic facing to determine the type and orientation of the fold.
6. explain how folds influence topography.
7. explain how to use cleavage surfaces to investigate folds.

Lesson 1: What Happens when Rocks Fold?

Several rather separate mechanical processes are involved in producing folds. Any given fold, or related system of folds, can form by a combination of these processes. Nonetheless, folding can be divided into two general types: flexural folding and passive folding.

Flexural folding is most important in strong but well-layered rocks, under low-temperature conditions; flexural folds are typical of low-grade metamorphic rocks. Passive folding becomes more important as the temperature increases and rocks more easily undergo internal distortion of their component materials. Passive folds are typical of higher grade metamorphic rocks.

Reading Assignment

• Read Davis, Reynolds, & Kluth: “Visual Impact” and “Mechanical Contradiction of Folding” (pp. 344-347)
• Read Davis, Reynolds, & Kluth: “Flexural Folding” (pp. 390-397)
• Read Davis, Reynolds, & Kluth: “Instability and Dominant Wavelength” (pp. 384-385)
• Read Davis, Reynolds, & Kluth: “Passive Folding” (pp. 401-403)

Study Questions

1. How is active folding different in a strong rock compared to a weak rock?
2. What structures develop within flexural-slip folds?
3. Buckling results in a layer that contains many small folds, but these small folds develop without the contacts of surrounding layers showing similar folds (Fig. 7.87, Davis, Reynolds, & Kluth, p. 396). How can only one layer be folded?
4. What fold profile characteristics indicate that passive folding has occurred?
5. What is the origin of passive folding in most cases?

Lesson 2: Fold Terminology

2.1. Anticlines and Synclines

A fold that is upward-closing (or convex-upward) is called an antiform (Fig. 7.12A and D, Davis, Reynolds, & Kluth, p. 352). The shape of the antiform allows it to act as a trap for petroleum. Since petroleum floats on groundwater, it rises upward if it is within permeable rocks (rocks through which fluids can flow). If an antiform is made of impermeable (tight) rocks, then rising petroleum will become trapped in the apex of the antiform (Fig. 5.1).

Figure 5.1. Antiform as petroleum trap

Downward-closing (or concave-upward) folds are called synforms (Fig. 7.12B & C, Davis, Reynolds, & Kluth, p. 352). An easy way to remember the difference between antiforms and synforms is that you can fit a capital letter “A” under an antiform, and that a synform “sinks” down in the middle. Antiforms and synforms generally occur side-by-side in fold trains.

Fold terminology distinguishes between folds that point in the way-up direction and folds that do not. In an anticline, the fold points in the way-up direction (in the direction of younger beds), whereas in a syncline, the fold points in the opposite direction. If strata are right-way-up, then an antiform will also be an anticline (Fig. 7.12A, Davis, Reynolds, & Kluth, p. 352), and a synform will also be a syncline (Fig. 7.12C). However, if strata are upside-down, then an antiform will point away from the way-up direction, it will be classified as a syncline (Fig. 7.12D), and it would be called an antiformal syncline. In upside-down strata, a synform points in the way-up direction, it is classified as an anticline (7.12C), and it would be called a synformal anticline (Fig. 7.12B).

Before you decide that memorizing the preceding paragraph will be easier than trying to sort “forms” from “clines,” consider this trick to help you with the distinction: form refers to the shape of the fold. Therefore, name the shape first (antiformal or synformal), then determine whether the fold points in the way-up direction (anticline) or not (syncline). Note that if you do not have information about stratigraphic facing, then you can only describe the shape of the fold (antiform or synform).

Reading Assignment

• Read Davis, Reynolds, & Kluth: “Anticlines and Synclines” (pp. 351-357)
• Read Davis, Reynolds, & Kluth: “Geometric Analysis of Folds” up to “Geometric Coordination of Hinge Lines and Axial Surfaces” (pp. 358-362)

2.2. Fold Anatomy

Reading Assignment

• Read Davis, Reynolds, & Kluth: “Geometric Analysis of Folds,” up to “Geometric Coordination of Hinge Lines and Axial Surfaces” (pp. 382-386)

Use the letters a through f, corresponding to the list below, to label the parts of the fold in Figure 5.2.

1. hinge point
2. hinge line
3. hinge zone
4. limb
5. inflection point
6. axial surface

Figure 5.2. The main parts of a folded surface

An important concept discussed in the readings above is the fold profile. A profile plane is any plane perpendicular to the fold axis, and the fold profile is the shape of the fold as seen in this plane (Fig. 11-8, Marshak & Mitra, p. 217). The fold profile is the true, two-dimensional shape of the fold. The shape of the fold as seen in a plane oriented any way other than perpendicular to the fold axis is misleading. To see why, consider the shape of a pencil: it is long in one direction (its axial direction), and round, or circular, as viewed end-on (the profile plane). If the pencil were cut obliquely to its length, the cut surface would be elliptical. The oblique view of the pencil gives a misleading idea of its true shape.

Study Questions

1. What is the difference between a fold that is upside-down and one that is overturned?
2. What does it mean to say that folds are superposed?

Lesson 3: How to Describe the Orientation of a Fold

3.1. Fold Description

The trend and plunge of the hinge line and the strike and dip of the axial surface describe the three-dimensional attitude of a fold. These measurements require that the fold hinge is a straight line, and that the axial surface is a plane. Many folds, however, have hinges that are not straight lines: if the hinge happens to wiggle up and down (e.g., Fig. 7.26C, Davis, Reynolds, & Kluth, p. 359) or side to side (e.g., Fig. 7.29C, Davis, Reynolds, & Kluth, p. 360), then we must describe segments of it for which the hinge line is reasonably straight.

In this course, we will focus on folds with straight hinge lines. Such folds are called cylindrical folds. More precisely, a cylindrical fold is one for which a folded surface can be generated by moving the straight hinge line parallel to itself (see Fig. 7.34, Davis, Reynolds, & Kluth, p. 363). Folds that do not satisfy this rule are non-cylindrical folds. The word cylindrical might bring to mind a circular cross-section, like that of a pipe or can, but the limbs of cylindrical folds are not limited in terms of their curvature. In other words, any fold profile that you can think of could belong to a cylindrical fold.

There are some subtleties in terminology when discussing folds. When referring to the actual parts of a fold, the terms hinge, hinge line, and so forth are used. When describing the orientation of a cylindrical fold, it is common to refer to the abstract geometrical structures that are used to approximate the hinge and axial surface of the fold. The imaginary line that approximates the hinge is called the fold axis. The axial surface is itself an imaginary surface, but when discussing the geometry of a cylindrical fold, it is referred to as an axial plane.

Reading Assignment

• Read Davis, Reynolds, & Kluth: “Geometric Coordination of Hinge Lines and Axial Surfaces” and “Cylindrical versus Noncylindrical Folds” (pp. 362-364)

A fold can have any three-dimensional orientation. This provides a convenient means of classification based on the dip of the axial plane and the plunge angle of the fold axis. This classification system is summarized by a Fleuty diagram, as seen in Figure 7.33 (Davis, Reynolds, & Kluth, p. 363).

The dip of the axial plane can be classified as upright, inclined, or recumbent:

• upright: the dip is 80°-90° (subvertical dip).
• inclined: the dip is 10°-80°. This class is further divided into
  1. gently inclined: 10°-30°
  2. moderately inclined: 30°-60°
  3. steeply inclined: 60°-80°
• recumbent: the dip is 0°-10° (subhorizontal dip).

The plunge angle of the fold axis can be classified as horizontal, plunging, or vertical:

• horizontal (or subhorizontal): the plunge is 0°-10°.
• plunging: the plunge is 10°-80°. This class is further divided into
  1. gently plunging: 10°-30°
  2. moderately plunging: 30°-60°
  3. steeply plunging: 60°-80°
• vertical (or subvertical): the plunge is 80°-90°.

The dip and plunge classifications can be combined to produce a single fold orientation classification. Examples of these are shown in Figures 5.3-5.5 (Study Guide). (Note that the dot-shaded plane (AP) is the axial plane, B is the hinge line, and the light lines (s.l.) on the bed are strike lines).

Figure 5.3 shows upright axial planes combined with the three plunge classes, resulting in folds that are

• horizontal upright;
• plunging upright (the fold shown is gently plunging); and
• vertical (a vertical hinge line, in which case the axial plane must also be vertical or upright).

Figure 5.3. Folds with upright axial planes

Figure 5.4 shows inclined axial planes combined with the only two possible plunge classes, resulting in folds that are

• horizontal inclined,
• plunging inclined (the fold shown is moderately plunging), and
• reclined. Reclined is a special case of plunging inclined wherein the hinge line lies parallel to the dip line of the axial plane (i.e., the dip and plunge angles have the same numerical values).

Figure 5.4. Folds with inclined axial planes

If the axial plane is horizontal (within 10°), then the hinge line must also be horizontal (within 10°). This produces a recumbent fold (Fig. 5.5). A recumbent fold with axial-plane dip of several degrees could have its hinge line parallel to the dip direction, in which case we can use the term reclined recumbent.

Figure 5.5. Recumbent fold with horizontal axial plane

Recumbent, vertical, and reclined folds cannot be called antiforms or synforms because they close sideways rather than upwards or downwards. As a result, they are sometimes called sideways-closing folds, or, more commonly, neutral folds.

In reality, no fold retains perfectly constant orientation throughout its entire length. Furthermore, all folds stop or die out eventually. Some common ways in which folds can change plunge are shown in Figure 11.18 (Marshak & Mitra, p. 223). The axial plane may or may not change in these cases. One common way for folds to die out is to become narrower and to change plunge in the way shown in the first two sketches of Figure 11.18.

The most complicated way for folds to undergo changes in orientation is called polyphase folding. This occurs where more than one system of folds have developed, such that early folds are themselves twisted or refolded by later superposed folds. In Figure 16.2 (Marshak & Mitra, p. 363), you can see that both axial planes and hinge lines take on a wide variety of orientations.

Polyphase folding affects the way-up of folded rocks. In Figure 5.6 (Study Guide) you can see the normal stratigraphic situation, where originally horizontal beds (Fig. 5.6a) are folded, and the rocks young (or face) away from the cores of antiforms (Fig. 5.6b), and toward the cores of synforms (Fig. 5.6c). Figure 5.7 shows an example of recumbent folds before (Fig. 5.7a) and after (Fig. 5.7b) undergoing a second phase of deformation. Take a moment to work through Figure 5.7b: determine the way-up for each of the identified segments, and verify for yourself that you understand why each segment has been named as it has.

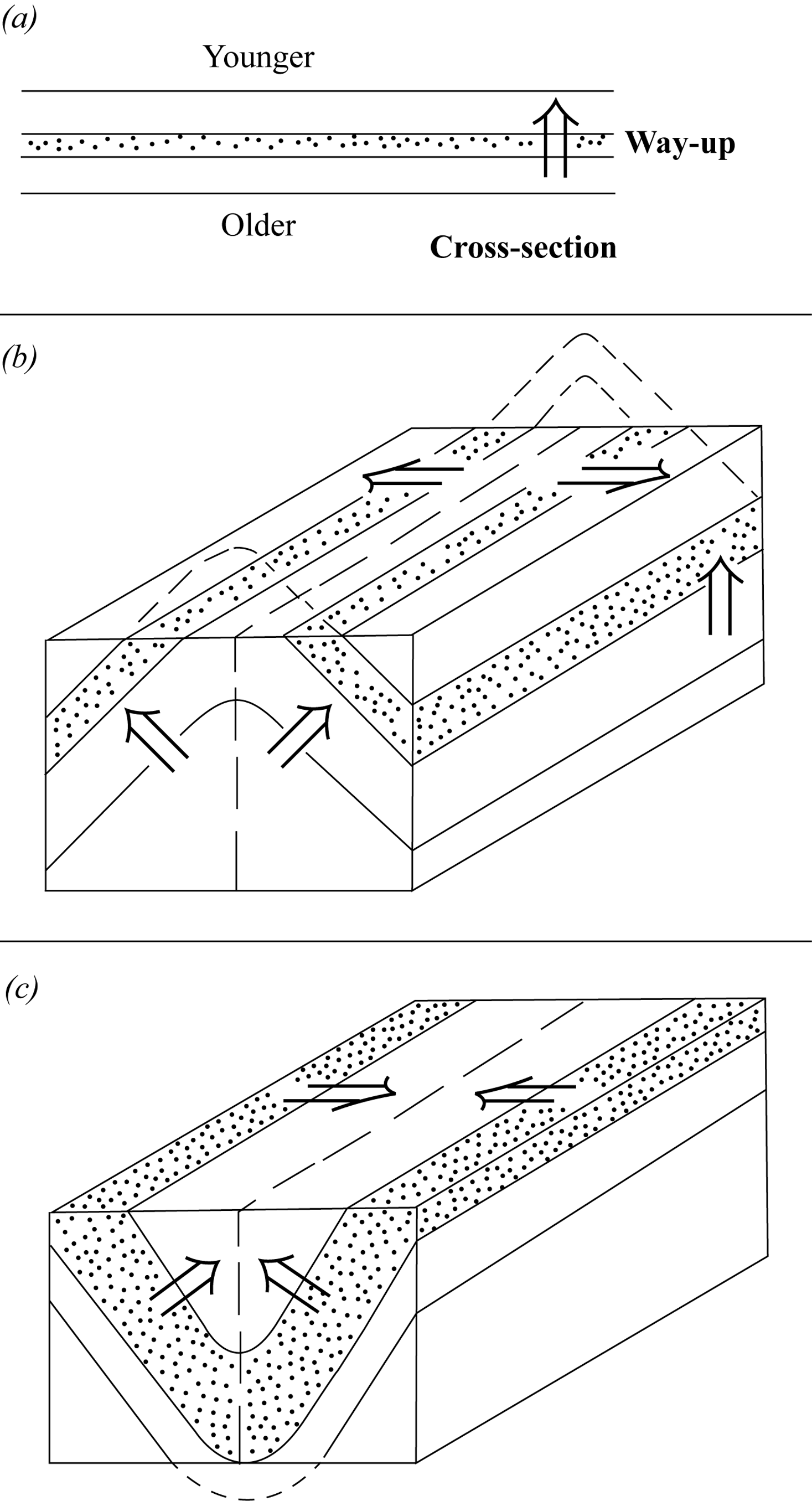
Figure 5.6. Normal stratigraphic facing in anticlines and synclines

Figure 5.7. Effects of polyphase folding on stratigraphic facing

3.2. Why Fold Orientation Is Important

In many areas of the world, understanding fold geometry has considerable economic importance—especially in prospecting and developing petroleum and mineral resources. Figure 5.8 (below) shows how misidentifying the dip of a fold's axial plane can lead to drilling in the wrong place, resulting in a considerable waste of money. In Figure 5.8 (a-c) there are a series of cross-sections 1 km wide across the region, and the plane of the cross-sections is also the fold profile plane.

Figure 5.8. Implications of fold geometry

The rocks exposed at the surface consist mainly of shale, with thin beds of sandstone (sh + ss). A geologist has measured a series of strikes and dips, indicated by the tick marks in Figure 5.8a. Elsewhere, in adjoining regions, thick beds of sandstone (ss.), shale (sh.) and limestone (ls.) underlie the interbedded shale and sandstone. The limestone bed is considered to be a likely host for petroleum.

The interpretation shown in Figure 5.8b assumes that an open, horizontal-upright fold is causing the change in dip directions on opposite sides of Y, and that the dip to the east of Z reflects an unrelated set of beds brought to the surface by a fault. This interpretation suggests that a drill-hole located at Y would intersect the crest of the fold in the limestone, and would enter the petroleum trap.

The dip lines can be interpreted differently, however (Fig. 5.8c): all the dips could be attributed to folding, with no fault present. In this case, the fold would be inclined, overturned to the east, and tightly closed. A drill-hole at Y would completely miss the antiformal crest in the limestone, missing the petroleum. For this fold, a hole should be drilled at X to hit the centre of the oil pool. Choosing between these two interpretations may be difficult, and could require additional information.

Study Questions

1. What is the difference between a recumbent fold and a reclined fold?
2. If a fold is horizontal upright, how is it oriented?
3. If a fold is vertical, how is it oriented?

Lesson 4: Folds in Outcrop

In Figure 5.9a (Study Guide) a plunging, upright antiform is truncated by a horizontal surface. The axial plane is shown in a dot pattern, the plunge angle is labelled “plunge,” and the trend, or direction, of plunge is shown by the arrow. The horizontal “outcrop” surface is shown in thin, horizontal-line shading. The outcrop pattern of this antiform is a curved line that approximates an arc of an ellipse. This is seen more clearly in map view (Figure 5.9b, left), where the outcropping edge of the axial plane is the axial trace (a.t.), and the direction of plunge is given by the arrow. The outcrop pattern for a plunging synform is also curved, but where the U-shape points down-plunge for an antiform, in a synform, the hinge line plunges towards the inside of the U-shaped outcrop pattern (Fig. 5.9b, right).

Complexities occur where the topographic surface is not flat, but consists of hills and valleys. We have seen already that complex, V-shaped outcrop patterns occur where uniformly dipping beds are truncated by a variable topographic surface. Where variable topographic surfaces truncate folded rocks, individual changes in outcrop direction can be caused either by topography or by fold hinge zones, and the resulting pattern can be difficult to decipher.

Complexities also occur where the fold is horizontal, inclined, reclined, vertical, or recumbent. We won't go into the details of all possible cases; instead, we will consider three situations involving flat, horizontal topography more fully.

First consider a horizontal fold, either upright or inclined. The beds involved in this fold will form straight outcrop patterns, with beds on opposite sides of the axial trace running parallel to each other. You can reproduce this situation with a piece of paper. First, fold the paper with the hinge line parallel to the length of the paper. Then, holding the fold in an antiformal position, place it on a table top, and observe that the limbs form parallel outcrop lines where they touch the table.

Figure 5.9. Outcrop patterns on a flat surface

Next, consider the way a vertical fold will crop out on a horizontal surface by holding the fold you made for the previous example in a vertical position, and placing it on the table top. Note that it forms a U-shaped outcrop pattern similar to that for a plunging fold, but that the plunge of the fold is straight into the table. This is a common situation in many of the world's orogens.

Now hold the fold in a reclined position, placing it as close to the table as possible. Mentally project the fold onto the table-top. Again, it forms a U-shaped outcrop pattern, but the hinge line plunges in a direction perpendicular to the axial trace. This contrasts with the upright folds shown in Figure 5.9b, where the plunge direction is parallel to the axial trace.

Study Question

1. On flat, horizontal terrane, you see a U-shaped outcrop pattern with rocks getting younger towards the inside of the U. The beds are right-way-up, and the U closes to the southwest. What can you say about the structure that made this outcrop pattern?

Lesson 5: Folds and Topography

Where the sedimentary or volcanic rock sequence consists of alternating resistant (or competent) and recessive (easily weathered) rocks, the more resistant layers commonly form curving ridges along the limbs and noses of folds (see Fig. 5.10a below, which shows the same fold geometry as Fig 5.9a). This is a common situation in many of the world's orogens.

In general, there are three main topographic expressions for folds:

• first-order topography: antiforms form hills or mountains, and synforms form valleys (topography l, Fig. 5.10b; Fig. 7.1, Davis, Reynolds, & Kluth, p. 345)
• second-order topography: the soft rocks have been eroded away in the antiformal cores, leaving the fold limbs to form hilly or mountainous ridges (topography 2, Fig. 5.10a & b)
• also second order topography: the resistant rock in a synform remains high after erosion of the surrounding softer materials (topography 3, Fig. 5.10b)

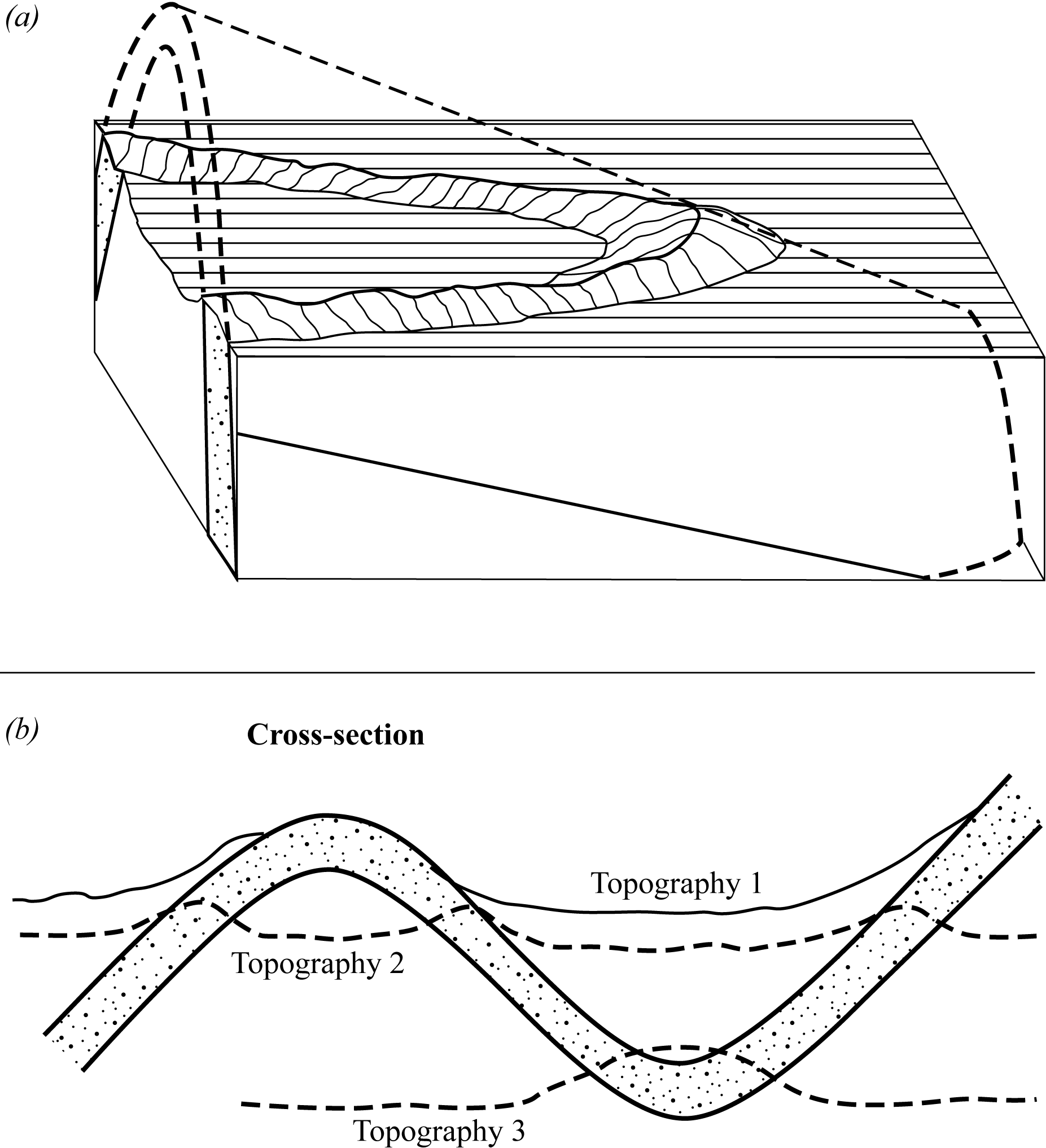
Figure 5.10. Topographic expressions for folds

Study Question

1. In Figure 5.10b, does the stippled bed represent a layer that is more resistant or less resistant to weathering than the surrounding rocks?

Assignment 5

You should now complete the theory portion of Assignment 5, which you can find in the assignment drop box. You will submit this assignment to your tutor for grading after you have also completed the lab portion.

Answers to Study Questions

Lesson 1

1. In strong rocks with well-defined beds, flexural-slip folding occurs. This folding is accommodated bylayer-parallel slippage, in which the beds slide along each other. This is similar to what happens when you fold a magazine with glossy pages. In weaker rocks in which the contacts between beds are not as well-defined, flexural-flow folding occurs, in which the material undergoes ductile deformation to accommodate folding. You can demonstrate this for yourself by stacking three layers of Play-Doh™ (each ~1 cm thick), and then folding the stack. The layers will tend to stick to each other (i.e., no slip along contacts), so folding is accommodated by ductile deformation within the layers.
2. On the outside of the fold, where layers must be stretched to make the fold (e.g., Fig. 7.79, Davis, Reynolds, & Kluth, p. 393), structures such as tension fractures, boudins, and normal faults can develop. On the inside of the fold, where layers must be compressed for folding to occur, cleavage, thrust faults, and buckling can develop.
3. Buckling develops in a layer that is relatively strong compared to the immediately adjacent layers. Buckling forms when rocks are being compressed (shortened) parallel to bedding. During compression, the strong layer “wrinkles” to make up for the reduced amount of space. The surrounding weaker layers deform to accommodate the wrinkling. As a result, the contacts of other beds above and below are not disturbed by the wrinkling of the stronger layer.
4. Passive folds are thickened at the hinge, and limbs get thinner moving away from the hinge (e.g., Class 2 and 3 folds in Fig. 7.64, Davis, Reynolds, & Kluth, p. 383), reflecting the fact that material has been removed.
5. In most cases, passive folds occur where buckling and flexural folding has already developed. Development of cleavage (by pressure solution) then deforms these structures into passive folds (e.g., Fig. 9.39, Davis, Reynolds, & Kluth, p. 489).

Lesson 2

1. A fold is upside-down if its stratigraphic facing has been completely reversed. Synformal anticlines and antiformal synclines are examples of folds that are upside-down. If a fold is overturned, only part of it has reversed stratigraphic facing. For example, an anticline can be tipped sideways to the point where one limb has its stratigraphic facing reversed, but the other does not.
2. If folds are superposed it means that one fold (or set of folds) formed, and then the second fold (or set of folds) deformed the rocks that were already folded.

Lesson 3

1. With both recumbent and reclined folds, the dip of the axial plane and the plunge of the fold axis have the same numerical values. The difference is that a recumbent fold has a horizontal fold axis and a horizontal axial plane, whereas a reclined fold is a special case of a plunging inclined fold.
2. A horizontal upright fold has a horizontal (0°-10°) fold axis and a vertical (80°-90°) axial plane.
3. A vertical fold has a vertical (80°-90°) fold axis and axial plane.

Lesson 4

1. The U-shaped pattern indicates that the structure is a plunging fold. The fact that the strata get younger toward the inside of the U means that the structure is a synform. The strata are right-way-up, so the structure is also a syncline. The fold closes to the southwest, so the hinge is plunging to the northeast.

Lesson 5

1. The stippled bed is more resistant to weathering than the surrounding rocks. Notice how it is higher than the other rocks in each of the topographic profiles.